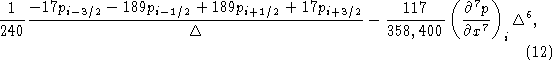
The ''left'' boundary point is computed by
The ''right'' boundary point has the similar formulation.
By requiring the minimum truncation error for the sixth-order difference
scheme we obtain the sixth-order compact scheme
![]()
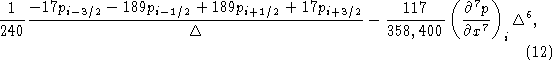
The ''left'' boundary point is computed by
![]()
![]()
The ''right'' boundary point has the similar formulation.
The last terms in (4) and (12) suggest that the compact scheme can provide
more accuracy. The error ratio between the sixth-order compact and ordinary
schemes is
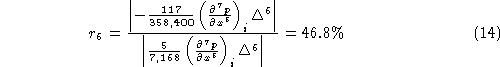
which means a more than 50% reduction of the truncation error when we use the
compact scheme for the 6th-order accuracy. We use the pressure gradient error
reduction in the ![]() -coordinate system as an example to illustrate the
benefit of using high-order compact difference schemes.
-coordinate system as an example to illustrate the
benefit of using high-order compact difference schemes.